a. \(y' = \dfrac{{\left( {\sin x} \right)'.x - \sin x.\left( {x'} \right)}}{{{x^2}}}\) \( + \dfrac{{x'\sin x - x.\left( {\sin x} \right)'}}{{{{\sin }^2}x}}\)
\(\eqalign{ & = {{x\cos x - \sin x} \over {{x^2}}} + {{\sin x - x\cos x} \over {{{\sin }^2}x}} \cr & = \left( {x\cos x - {\mathop{\rm sinx}\nolimits} } \right)\left( {{1 \over {{x^2}}} - {1 \over {{{\sin }^2}x}}} \right) \cr} \)
b. 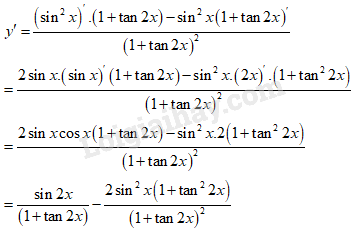
c. \(y' = \left( {\sin x} \right)'.\dfrac{1}{{{{\cos }^2}\left( {\sin x} \right)}}\) \( \displaystyle = {{\cos x} \over {{{\cos }^2}\left( {\sin x} \right)}}\)
d. \(y' = x'.\cot \left( {{x^2} - 1} \right) + x.\left[ {\cot \left( {{x^2} - 1} \right)} \right]'\) \( = \cot \left( {{x^2} - 1} \right) + x.\left( {{x^2} - 1} \right)'.\dfrac{{ - 1}}{{{{\sin }^2}\left( {{x^2} - 1} \right)}}\)
\(\eqalign{ & = \cot \left( {{x^2} - 1} \right) + x.{{ - 2x} \over {{{\sin }^2}\left( {{x^2} - 1} \right)}} \cr & = \cot \left( {{x^2} - 1} \right) - {{2{x^2}} \over {{{\sin }^2}\left( {{x^2} - 1} \right)}} \cr} \)
e. 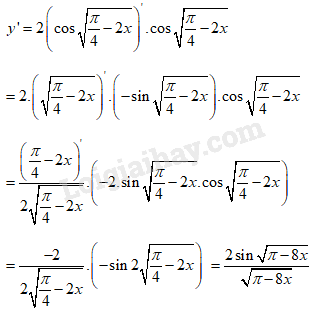
f. \(y' = x'\sqrt {\sin 3x} + x.\left( {\sqrt {\sin 3x} } \right)'\) \( = \sqrt {\sin 3x} + x.\dfrac{{\left( {\sin 3x} \right)'}}{{2\sqrt {\sin 3x} }}\) \( \displaystyle = \sqrt {\sin 3x} + x.{{3\cos 3x} \over {2\sqrt {\sin 3x} }} \) \(\displaystyle = {{2\sin 3x + 3x\cos 3x} \over {2\sqrt {\sin 3x} }}\)