Bài 3.31 trang 178 SBT giải tích 12

Tính diện tích hình phẳng giới hạn bởi các đường sau:

a) \(\displaystyle  y = 2x - {x^2},x + y = 2\);

b) \(\displaystyle  y = {x^3} - 12x,y = {x^2}\);

c) \(\displaystyle  x + y = 1;x + y =  - 1;\) \(\displaystyle  x - y = 1;x - y =  - 1\);

d) \(\displaystyle  y = \frac{1}{{1 + {x^2}}},y = \frac{1}{2}\)

e) \(\displaystyle  y = {x^3} - 1\) và tiếp tuyến với \(\displaystyle  y = {x^3} - 1\) tại điểm \(\displaystyle  \left( { - 1; - 2} \right)\).

a) \(\displaystyle  y = 2x - {x^2},x + y = 2\)

Ta có: \(\displaystyle  y = 2x - {x^2},y = 2 - x\)

Phương trình hoành độ giao điểm: \(\displaystyle  2x - {x^2} = 2 - x\) \(\displaystyle   \Leftrightarrow {x^2} - 3x + 2 = 0 \Leftrightarrow \left[ \begin{array}{l}x = 1\\x = 2\end{array} \right.\)

Khi đó diện tích \(\displaystyle  S = \int\limits_1^2 {\left| {2x - {x^2} - 2 + x} \right|dx} \) \(\displaystyle   = \int\limits_1^2 {\left| { - {x^2} + 3x - 2} \right|dx} \) \(\displaystyle   = \int\limits_1^2 {\left( { - {x^2} + 3x - 2} \right)dx} \)

\(\displaystyle   = \left. {\left( { - \frac{{{x^3}}}{3} + \frac{3}{2}{x^2} - 2x} \right)} \right|_1^2\) \(\displaystyle   =  - \frac{8}{3} + 6 - 4 + \frac{1}{3} - \frac{3}{2} + 2 = \frac{1}{6}\)

Vậy \(\displaystyle  S = \frac{1}{6}\).

b) \(\displaystyle  y = {x^3} - 12x,y = {x^2}\)

Phương trình hoành độ giao điểm:

\(\displaystyle  {x^3} - 12x = {x^2}\)\(\displaystyle   \Leftrightarrow {x^3} - {x^2} - 12x = 0\) \(\displaystyle   \Leftrightarrow x\left( {{x^2} - x - 12} \right) = 0\) \(\displaystyle   \Leftrightarrow \left[ \begin{array}{l}x = 0\\{x^2} - x - 12 = 0\end{array} \right.\) \(\displaystyle   \Leftrightarrow \left[ \begin{array}{l}x = 0\\x =  - 3\\x = 4\end{array} \right.\)

Diện tích là:

\(\displaystyle  S = \int\limits_{ - 3}^4 {\left| {{x^3} - 12x - {x^2}} \right|dx} \) \(\displaystyle   = \int\limits_{ - 3}^0 {\left| {{x^3} - 12x - {x^2}} \right|dx} \) \(\displaystyle   + \int\limits_0^4 {\left| {{x^3} - 12x - {x^2}} \right|dx} \)

\(\displaystyle   = \left| {\int\limits_{ - 3}^0 {\left( {{x^3} - {x^2} - 12x} \right)dx} } \right|\) \(\displaystyle   + \left| {\int\limits_0^4 {\left( {{x^3} - {x^2} - 12x} \right)dx} } \right|\) \(\displaystyle   = \frac{{99}}{4} + \frac{{160}}{3} = \frac{{937}}{{12}}\).

Vậy \(\displaystyle  S = \frac{{937}}{{12}}\).

c) \(\displaystyle  x + y = 1;x + y =  - 1;\) \(\displaystyle  x - y = 1;x - y =  - 1\);

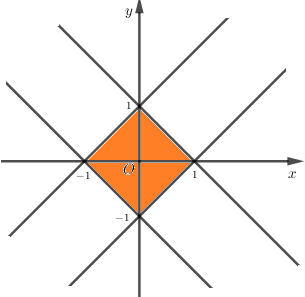
Vẽ các đường thẳng \(\displaystyle  x + y = 1;x + y =  - 1;\) \(\displaystyle  x - y = 1;x - y =  - 1\) trên hệ tục tọa độ ta được phần cần tính diện tích là hình vuông có các đỉnh \(\displaystyle  \left( { - 1;0} \right),\left( {0; - 1} \right),\left( {1;0} \right),\left( {0;1} \right)\).

Diện tích hình vuông là: \(\displaystyle  S = 4.\frac{1}{2}.1.1 = 2\).

Chú ý:

Sử dụng công thức tích phân ta được \(\displaystyle  S = 4\int\limits_0^1 {\left( {1 - x} \right)dx} \)\(\displaystyle   = 4\left. {\left( {x - \frac{{{x^2}}}{2}} \right)} \right|_0^1 = 4\left( {1 - \frac{1}{2}} \right) = 2\).

d) \(\displaystyle  y = \frac{1}{{1 + {x^2}}},y = \frac{1}{2}\)

Ta có: \(\displaystyle  \frac{1}{{1 + {x^2}}} = \frac{1}{2}\)\(\displaystyle   \Leftrightarrow 1 + {x^2} = 2\) \(\displaystyle   \Leftrightarrow {x^2} = 1 \Leftrightarrow x =  \pm 1\).

Diện tích: \(\displaystyle  S = \int\limits_{ - 1}^1 {\left| {\frac{1}{{1 + {x^2}}} - \frac{1}{2}} \right|dx} \)\(\displaystyle   = \int\limits_{ - 1}^1 {\left( {\frac{1}{{1 + {x^2}}} - \frac{1}{2}} \right)dx} \)

Dễ thấy hàm số \(\displaystyle  y = \frac{1}{{{x^2} + 1}} - \frac{1}{2}\) là hàm số chẵn nên \(\displaystyle  S = \int\limits_{ - 1}^1 {\left( {\frac{1}{{1 + {x^2}}} - \frac{1}{2}} \right)dx} \) \(\displaystyle   = 2\int\limits_0^1 {\left( {\frac{1}{{1 + {x^2}}} - \frac{1}{2}} \right)dx} \)

Xét \(\displaystyle  I = \int\limits_0^1 {\left( {\frac{1}{{1 + {x^2}}} - \frac{1}{2}} \right)dx} \)\(\displaystyle   = \int\limits_0^1 {\frac{{dx}}{{1 + {x^2}}}}  - \frac{1}{2}\int\limits_0^1 {dx} \) \(\displaystyle   = J - \frac{1}{2}\) với \(\displaystyle  J = \int\limits_0^1 {\frac{1}{{1 + {x^2}}}dx} \)

Đặt \(\displaystyle  x = \tan t \Rightarrow dx = \left( {1 + {{\tan }^2}t} \right)dt\) \(\displaystyle   \Rightarrow J = \int\limits_0^{\frac{\pi }{4}} {\frac{{1 + {{\tan }^2}t}}{{1 + {{\tan }^2}t}}dt}  = \frac{\pi }{4}\)\(\displaystyle   \Rightarrow I = \frac{\pi }{4} - \frac{1}{2}\)

Vậy \(\displaystyle  S = 2I = 2.\left( {\frac{\pi }{4} - \frac{1}{2}} \right) = \frac{\pi }{2} - 1\).

e) \(\displaystyle  y = {x^3} - 1\) và tiếp tuyến với \(\displaystyle  y = {x^3} - 1\) tại điểm \(\displaystyle  \left( { - 1; - 2} \right)\).

Xét \(\displaystyle  y = g\left( x \right) = {x^3} - 1\) có \(\displaystyle  g'\left( x \right) = 3{x^2}\)\(\displaystyle   \Rightarrow g'\left( { - 1} \right) = 3\).

Phương trình tiếp tuyến của đồ thị hàm số \(\displaystyle  y = g\left( x \right)\) tại điểm \(\displaystyle  \left( { - 1; - 2} \right)\) là:

\(\displaystyle  y = 3\left( {x + 1} \right) - 2\) hay \(\displaystyle  y = 3x + 1\).

Xét phương trình \(\displaystyle  {x^3} - 1 = 3x + 1 \Leftrightarrow {x^3} - 3x - 2 = 0\) \(\displaystyle   \Leftrightarrow \left( {x - 2} \right){\left( {x + 1} \right)^2} = 0 \Leftrightarrow \left[ \begin{array}{l}x =  - 1\\x = 2\end{array} \right.\)

Diện tích: \(\displaystyle  S = \int\limits_{ - 1}^2 {\left| {{x^3} - 3x - 2} \right|dx} \) \(\displaystyle   = \int\limits_{ - 1}^2 {\left( { - {x^3} + 3x + 2} \right)dx} \) \(\displaystyle   = \left. {\left( { - \frac{{{x^4}}}{4} + \frac{3}{2}{x^2} + 2x} \right)} \right|_{ - 1}^2\) \(\displaystyle   =  - 4 + 6 + 4 + \frac{1}{4} - \frac{3}{2} + 2 = \frac{{27}}{4}\).

Vậy \(\displaystyle  S = \frac{{27}}{4}\).