Trong các phân số đã cho, phân số bằng phân số \(\displaystyle - {{16} \over {24}}\) là \(\displaystyle{{ - 2} \over 3}.\)
Chọn đáp án (C.)
Bài 2.2
Các cặp phân số bằng nhau là :
(A) \(\displaystyle{{ - 3} \over 4}\) và \(\displaystyle{{ - 4} \over 3}\); (B) \(\displaystyle - {2 \over 3}\) và \(\displaystyle{6 \over 9}\);
(C) \(\displaystyle{3 \over 7}\) và \(\displaystyle{{ - 3} \over 7}\); (D) \(\displaystyle{7 \over 8}\) và \(\displaystyle{{ - 35} \over { - 40}}\)
Hãy chọn câu trả lời đúng.
+) \(\displaystyle{{ - 3} \over 4}\ne \displaystyle{{ - 4} \over 3}\) vì \((-3). 3 \ne 4. (-4).\)
+) \(\displaystyle - {2 \over 3}\ne \displaystyle{6 \over 9}\) vì \(-\dfrac{2}{3} = \dfrac{-2}{3}\)và \((-2).9 \ne 3.6 .\)
+) \(\displaystyle{3 \over 7} \ne\displaystyle{{ - 3} \over 7}\) vì \( 3.7\ne 7.(-3)\)
+) \(\displaystyle{7 \over 8} = \displaystyle{{ - 35} \over { - 40}}\) vì \( 7.(-40) = 8. (-35).\)
Chọn đáp án (D).
Bài 2.3
Hãy tìm các số nguyên \(x\) và \(y\), biết :
\(\displaystyle{{ - 2} \over x} = {y \over 3}\) và \(x < 0 < y.\)
Ta có \(\displaystyle{{ - 2} \over x} = {y \over 3}\)
\(\Rightarrow x.y = -2.3 = -6.\)
Vì \(x < 0 < y\) nên ta có bảng sau :
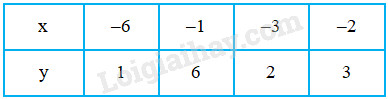
Bài 2.4
Tìm các số nguyên \(x\) và \(y\), biết :
\(\displaystyle{{x - 3} \over {y - 2}} = {3 \over 2}\) và \(x – y = 4\)
\(\displaystyle{{x - 3} \over {y - 2}} = {3 \over 2}\) nên \(2(x \,– 3) = 3(y\, – 2)\)
\( \Rightarrow 2x – 6 = 3y – 6\) \(\Rightarrow 2x = 3y.\)
Ta có : \(3y-2y=y\)
\(\Rightarrow 2x – 2y = y\) hay \(2(x – y) = y\)
\(\Rightarrow y = 2 (x-y)=2.4 = 8.\)
Vậy \(\displaystyle y = 8\;;\;x = {{3y} \over 2} = {{3.8} \over 2} = 12.\)