a) Ta có: \(y' = (a - 1){x^2} + 2ax + 3a - 2\).
+) Với \(a = 1,y' = 2x + 1\;\) đổi dấu khi \(x\) đi qua \( - \dfrac{1}{2}\). Hàm số không luôn luôn đồng biến.
+) Với \(a \ne 1\) thì với mọi \(x\) mà tại đó \(y' \ge 0\)\( \Leftrightarrow \left\{ \begin{array}{l}a - 1 > 0\\\Delta ' = - 2{a^2} + 5a - 2 \le 0\end{array} \right.\)\( \Leftrightarrow \left\{ \begin{array}{l}a > 1\\\left[ \begin{array}{l}a \ge 2\\a \le \dfrac{1}{2}\end{array} \right.\end{array} \right.\) \( \Leftrightarrow a \ge 2\)
(khi \(a = 2\) thì \(y' = 0\;\) chỉ tại \(x = - 2\))
Vậy với \(a \ge 2\) hàm số luôn luôn đồng biến.
b) Đồ thị cắt trục hoành tại ba điểm phân biệt khi và chỉ khi phương trình \(y = 0\) có ba nghiệm phân biệt. Ta có:
\(y = 0\)\( \Leftrightarrow x\left[ {\dfrac{{(a - 1){x^2}}}{3} + ax + 3a - 2} \right] = 0\)
\( \Leftrightarrow x\left[ {(a - 1){x^2} + 3ax + 9a - 6} \right] = 0\)\( \Leftrightarrow \left[ \begin{array}{l}x = 0\\\left( {a - 1} \right){x^2} + 3ax + 9a - 6 = 0\,\left( * \right)\end{array} \right.\)
\(y = 0\) có ba nghiệm phân biệt khi và chỉ khi phương trình \(\left( * \right)\) có hai nghiệm phân biệt khác \(0\).
\( \Leftrightarrow \left\{ \begin{array}{l}a - 1 \ne 0\\\Delta > 0\\P \ne 0\end{array} \right.\) \( \Leftrightarrow \left\{ \begin{array}{l}a - 1 \ne 0\\9{a^2} - 4(a - 1)(9a - 6) > 0\\9a - 6 \ne 0\end{array} \right.\) \( \Leftrightarrow \left\{ \begin{array}{l}a \ne 1\\\dfrac{{10 - 2\sqrt 7 }}{9} < a < \dfrac{{10 + 2\sqrt 7 }}{9}\\a \ne \dfrac{2}{3}\end{array} \right.\)
Vậy \(a \in \left( {\dfrac{{10 - 2\sqrt 7 }}{9};\dfrac{{10 + 2\sqrt 7 }}{9}} \right)\backslash \left\{ {1;\dfrac{2}{3}} \right\}\).
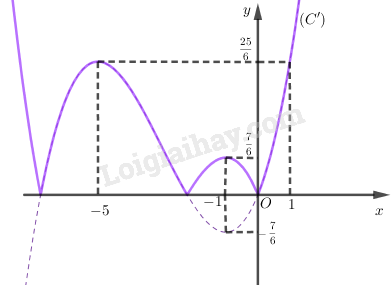
c) Khi \(a = \dfrac{3}{2}\) thì \(y = \dfrac{{{x^3}}}{6} + \dfrac{{3{x^2}}}{2} + \dfrac{{5x}}{2}\)
Ta có: \(y' = \dfrac{{{x^2}}}{2} + 3x + \dfrac{5}{2}\);\(y' = 0 \Leftrightarrow {x^2} + 6x + 5 = 0 \Leftrightarrow \left[ \begin{array}{l}x = - 1\\x = - 5\end{array} \right.\)
Bảng biến thiên:

Đồ thị:

Từ đồ thị hàm số \(y = \dfrac{{{x^3}}}{6} + \dfrac{{3{x^2}}}{2} + \dfrac{{5x}}{2}\) ta suy ra ngay đồ thị hàm số \(y = \left| {\dfrac{{{x^3}}}{6} + \dfrac{{3{x^2}}}{2} + \dfrac{{5x}}{2}} \right|\) như sau: